CANNONS!

One of the most recognizable structures in human history, the cannon is considered by some to be the pinnacle of warfare artillery. However, behind its thick shell and stern reputation lies an engineering marvel. It might seem strange, but physics is the backbone of the cannon.

A complicated cannon; assembled in a manner so that it remains hidden behind a barricade

As a project, our class will be building cannons on Thursday. The materials are a maximum of 5 soda cans, duct tape, and two Styrofoam cups. First of all, I believe that the cannon will be more successful if we make sure the inner part of the cannon is very smooth. This reduces the amount of friction that the cannon ball will experience. Also, the cannon barrel should be quite long, instead of very short. This way, the cannon ball will not experience as much air resistance when it leaves the cannon. Regarding the cannon ball itself, it might not actually have to be a ball. Looking at fast airplanes and cars, it seems that the most prevalent form of aerodynamic structure is a pointed tip. For example, F15 fighter jets are designed so that they are highly aerodynamic. The reason that these airplanes are so aerodynamic is because of their shape. When moving through the air, air resistance will be evenly distributed along the airplane rather than hitting it with a very strong force. (Newton's third law: every action has an opposite and equal reaction).

F15 Silent Eagle fighter jet

Now, for the angle of the cannon. In the kinematics unit, we learned about projectile motion. We learned the equation:

In this equation, d is the horizontal distance traveled, v is the velocity of the projectile and g is the force of gravity. From looking at this equation, we can already determine that the largest angle at which the cannon can be fired is 90°. This is because anything greater than that will result in a cannon that shoots straight upward or backwards (in other words a cannon that is not fit for combat). Thus, the angle must be less than 90°. But what angle will allow us to maximize the distance traveled? This is where the equation comes into place. 

Isolate sin2theta.

Using this equation, another clue is found. It appears that if theta = 45°, then the d will be be maximized. 
Since sin (45x2) = sin 90=1, therefore 45° will likely produce the farthest distance traveled.

This is because if theta=45°, then 2xtheta would equal 90°.

 

Newton's Problems

Lately we have been doing some extensive work on an interesting fellow called Sir Isaac Newton.

 

First of all, who is Newton? According to Encylcopedia Britannica, Newton was a English physicist, mathematician, astronomer and philosopher. He is also considered by many people to be one of the most influential people in human history. Throughout his lifetime, he discovered many new things and created new scientific laws. Among which were his three laws of motion:

1st Law: Inertia
Inertia is a term used by people to describe the tendency for an object to resist a change in motion. In everyday terms, the 1st law can be basically simplified into this: an object at rest will stay at rest, forever, as long as nothing pushes or pulls on it. An object in motion will stay in motion, traveling in a straight line, forever, until something pushes or pulls on it. In other words, objects will maintain their motion stationary or moving unless there is an external force. For example, beginner ice skaters find it difficult to stop their motion, unless they crash or fall.

2nd Law: F, m, a
Newton found that force, mass, and acceleration are all related. His second law states that: the acceleration of an object depends inversely on its mass and directly on the unbalanced force applied to it. This creates the equation:

3rd Law: Action=Reaction

Newton's third law applies to an action and a reaction. His law states that: for every action, there is an equal & opposite reaction. Take for example a a person standing on the ground. He is not moving vertically at all. Why does this happen? It happens because there are two forces acting on it and they are equal. First, gravity is applied to the person. However, there is a force that is opposite to gravity operating at the same intensity: the normal force. The third law applies.




Newton Problems
Newton's three laws apply to a plethora of things in this universe. As a result, it is only natural that questions and problems are formed. In this unit, we focus on four types of Newton Problems: Equilibrium, Incline (kinetic and static), Pulley, and Train.

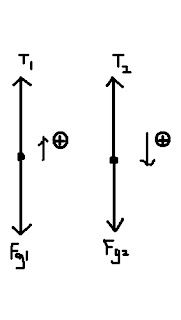
Equilibrium

Assumptions

-no friction

-set positive axes

-Tix=Tiy

a=0            

F= ma 
X component
Fₓ = ma                                                                                 

T1ₓ - T2ₓ = 0                                               

T1ₓ = T2ₓ      

Y component  

Fy = ma   
-Fg + T1y + T2y = 0
T1y + T2y = Fg

T1sinB + T2sinA = mg

 (T1sinB + T2sinA) / g = m

 

Inclines

STATIC(object not moving)

Assumptions

- fs = µFn

- a = 0

- +ve axes in the direction of decline
- no air resistance

 

KINETIC(object moving)

Assumptions

- fk = µkFn

- aₓ ≠ 0, ay = 0
- +ve in the direction of a

- no air resistance

 

F=ma

 Y component

Fy = ma
Fy = 0
Fn - Fgy = 0
Fn = Fgy
Fn = Fgcosθ
Fn = mgcosθ
X component

Fₓ = maₓ      

Fgₓ - f = maₓ

Fgsinθ - µFn = maₓ        

mgsinθ - µmgcosθ = maₓ

(mgsinθ - µmgcosθ) / m = aₓ

 

Pulleys

Assumptions

- frictionless pulleys + rope

- no air resistance

- multiple FBDs

- positive in direction of a

- T1 = T2

- a of the system is the same

 

to find m1

F = ma

Fₓ = ma

Fₓ = 0

Fy = m1ay

m1g - T = m1a (1)

to find m2

F = ma

Fₓ = ma

Fₓ = 0

Fy = m2ay

T - m2g = m2ay (2)

Find a

From (1) T = m1g - m1a    (3)

From (2) T = m2a + m2g   (4)

m1g - m1a = m2a + m2g

m1g - m2g = m2a + m1a

m1g - m2g = a (m2 + m1)

(m1g - m2g) / (m2 + m1) = a

 

Simplified FBD of train system (applies only to a)

Trains

Assumptions

- 1 FBD for a

- 3 FBDs for T1 and T2

- ay = 0

- a is consistent

- no air resistance
- weightless cables

- positive in direction of a

 

F = ma

X component

Fₓ = maₓ

Fₐ - f = maₓ 

Fₐ - µmg = maₓ

(Fₐ - µmg) / m = aₓ

Y Component
Fy = may
Fy = 0
Fn - mg = 0
Fn = mg

Projectile Motion

Projectile motion is one of the most important yet basic studies in physics. It can be described as a collaboration between the Big 5 problems and vector components. This is because it is recommended that these complicated questions be simplified by splitting the values into x and y components. (Knowledge of trigonometry is also recommended/needed.) There are 4 main types of projectile motion questions that you will likely encounter in Grade 11 physics:

Type 1:

In this case, an object starts off above its destination, and due to the force of gravity it falls. In most cases, the acceleration in y, or gravity, is 9.81. In type 1 questions, there is no acceleration for x, since there is no air resistance or opposing horizontal force. Therefore, Vx is also constant. In most scenarios for type 1 questions, the time is found first. Then, by substituting the time into an equation, other variables can be found, most often the distance in x or y.

Type 2

In type 2 questions, the acceleration in x is 0, like in type 1 questions. Also, there is gravity, but it is negative. Usually, and angle is given. Then, using sin and cos, the initial horizontal and vertical velocities can be found. To calculate the initial horizontal velocity, use the equation V1cos(theta). To calculate the initial vertical velocity, use the equation V1sin(theta). Also, in type 2 questions, R represents the horizontal distance and h represents the the maximum height. The unique part of type 2 questions is that dy is 0. As you can see from the diagram, the projectile begins and ends at ground level.

Type 3

In type 3 questions, everything is almost identical
to the type 2 questions. However, in this case, the projectile does not end at ground level. In this case, it landed on something. This object has a height, and usually it is this variable that we must calculate. Keep in mind that the height of this object is the dy, and it is positive. An example of a type 3 question is whether a baseball thrown at a certain angle will be able to pass a 3m tall object 15m away.

Type 4

In type 4 questions, everything is identical to type 3 questions except for one thing: now, the projectile is fired from a height that is higher than its estimated landing position. In this case, the dy is negative, because it is lower than the starting position. An example of a type 4 question is how far a rock will go if thrown at an angle from a 200m cliff.


Now that you know the four main types of projectile motion problems, there is only one thing you can do: Practice!

If you need help, you can use this website: http://cnx.org/content/m13856/latest/